UCEED 18 NAT (SECTION A ) SOLUTIONS
UCEED 18 paper is divided into three sections, A, B and C.
Section A (80 Marks) contains a total of 20 Numerical Answer Type (NAT) questions.
Section B (100 Marks) contains a total of 25 Multiple Select Questions (MSQ).
Section C (120 Marks) contains a total of 40 Multiple Choice Questions (MCQ).
IN THIS POST UCEED 18 NAT (SECTION A ) SOLUTIONS ARE GIVEN
Q.01 How many different types of characters appear in the figure given below?
ANS 21 as shown
Q.02 Given below is a series of numbers. Which number will replace the question mark?
101, 103, 107, 109, ?, 127, 131, 137, 139, 149, 151, 157, 163
ANS 113
These are all prime numbers ,therefore 113 replaces
Q.03 How many lines are there in the image given below?
ANS There are 22 lines.
You can count
- direction wise - horizontal,vertical,inclined
- length wise - small, medium, large
Q.04 How many configurations of blocks appear only once in the figure given below?
ANS 12 blocks.
Observe it .then cross check it i.e. there are 20 blocks and 4 blocks are repeated so
20 - (4 x 2) = 12
Q.05 What is the number of fonts used in the words given below?
ANS Letter "a" is common in all words so we can easily compare and find the number of fonts easily.
here there are 6 fonts.
Q.06 What is the maximum number of whole cuboids of length = 2 cm, breadth = 1 cm and height
= 1 cm that can be packed into a larger cuboid of length = 3 cm, breadth = 3 cm and height = 11 cm?
ANS volume of large cuboid = L x B x H= 3 x 3 x 11 =99 cm3
volume of small cuboid = 2 x 2 x 1 =2 cm3
Let number of small cuboids be N that can be packed into a larger cuboid
then N x 2=99
N = 49.5 i.e. 49 numbers
Alternately ,you can find as shown in figure
arrange small cuboid we see that in 2 cm ht 9 cuboids can be placed (1,2,3,4) X 2 i.e 8 nos horizontally and ( 5) X 1 vertically. In 10 cm ht there will be 9 x 5 = 45 cuboid In remaining 1 cm ht there will be 4 cuboids Total 49 cuboids.
Q.07 Prof. Paittiyam, in an attempt to create the world’s most delicious dish, managed to merge a
cylindrical Idli (diameter = 17 cm, height = 6 cm) with a torus shaped medu wada (inner hole diameter
= 2 cm, outer diameter = 16 cm, and tube diameter i.e height of torus = 7 cm) such that the medu
wada is parallel to the base of the Idli and the centeroids of the two solids coincide. How many surfacesdoes the resulting solid contain?
Centroid of cylinder and torus is in centre . As the dia of torus is less than cylinder but height is more therefore torus will protrude from both ends of the cylinder for fulfulling the condition that the centroids of the two solids coincide as shown in figure . The resultant solid will have 7 surfaces.
Note that outer and inner side of the torus will be counted once only.
Q.08 What is the maximum number of equilateral triangles (side 3 cm) that can be placed in a
square of side 6.8 cm without overlapping each other?
as shown there will be 8 equilateral triangles.
Q.09 How many triangles are there in the figure shown below?
In triangle abc there are these triangles
abc , abh , ach , bgc , aec , afb , bec , bfc ,
agb , agc , aeg , agf , egb , bgh , ghc , cgf
total 16 triangles
similarly in triangle bcd there will be 16 triangles
combining both triangles there are these triangles in addition
abd , adc , bgl , cgl , abl , acl , bgd , dgc
total 8 nos.
ANS so grand total , 16 + 16 + 8 = 40 triangles
Q 10 . Count the number of stacked triangular pieces in the image shown below.
A. Count the number of columns and pieces respectively.
As shown the total number of stacked triangular pieces = 46 .
Q.11 Shown below is a combination of fixed pulleys. If the triangular load moves down, how many
pulleys will rotate in the same direction as the smallest pulley (including itself)? The overlapping pathsdo not interfere with each other.
ANS directions of pulleys are shown in figure, and smallest pulley is indicated as "s" it rotates anticlockwise and there are 11 such pulleys which rotates anticlockwise.
Q.12 The current time is 10 minutes past 3 in the afternoon. What will the angle be between the
hour hand and the minute hand of the clock, 4 hours and 40 minutes from now?
ANS The current time is 03:10 pm ,the time after 4 hrs and 40 min will be 07:50 pm.
the angle between hour hand and minute hand can be found by the formula
x = ( 60 h-11 m)/2
where h = hours( h<12)
m = minutes (m<60)
x = angle measure between hour hand and minute hand of a clock at a given time
so, at 07:50
x = (60 x7 - 11x50) /2 = 65 degrees
Q.13 The word ‘Ape’ is an anagram of ‘Pea’. How many of the following words are anagrams of
animals?
Glow, flow, loin, sit, balm, bare, god, lane, act, tab, bit, tar, reef
ANS wolf , loin, lamb, bear ,dog ,cat, bat, rat, ( total 8 words)
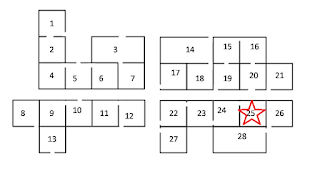
Q.14 Privacy of a space depends on the level of closure it has from its surroundings i.e. the difficulty
of accessing that space from outside the house. The figure below shows the plans of four houses at a
village street junction. Which is the most private room amongst all the rooms across all the houses?
ANS as we see that room no 25 is most difficult to access. one has to cross room no 22,23,and 24 to go to that room.
other rooms are either directly accessible or through one room.
Q.15 The figure below shows the layout plan of the conservative Themmalwadi village which
consists of a cluster of houses. Black lines represent walls and the openings in those walls represent
either windows or doors. Also marked are the houses of Riya (who lives with her family) and Barkya,
who are in love and having an affair. So, hidden from the eyes of the villagers, they regularly meet at
night in Barkya’s house. Themmalwadi is so conservative that even the sight of women on the street
at night raises eyebrows. If Riya takes the path that has the least number of openings, what is the
number of openings that she will have to pass by to get to Barkya’s house?
ANS Route shown in green will have minimum 3 openings that Riya has to pass by to get to Barkya's house .
Q.16 Shown in the figure is a pyramid with a square base, formed by vertices EFGH and a top vertex
I. The diagonally opposite vertices, EG and FH are connected to form the vertex J at their intersection.
The vertices I and J are also connected as shown. How many triangles are formed by all these vertices?
ANS Triangles formed by all these vertices are
EIF , EIH , HIG , FIG , EFJ , EJH , HJG , FJG
EJI , HJI , GJI , FJI , IFH , IEG , EGH , EFH , FEG , FHG
TOTAL 18 Triangles
Q.17 The length and breadth of the white rectangle are 6 and 4 respectively. The proportions of the
outer black, middle red and inner white rectangles are the same. What is the length of the hypotenuse
of triangle ABC?
ANS Black ,red, and white rectangles are in same proportion and as we can see in the figure that middle red rectangle is double the white so its length & breadth will be 12 and 8 respectively.
Black rectangle is double the red so its length and breadth will be 24 and 16 respectively.
But we have to find the hypotenuse of triangle ABC ,and AC is half of the length of black rectangle i.e.AC =12 and BC = 16
by Pythagoras theorem AB x AB = (AC x AC) + (BC x BC)
AB x AB = (12 x 12) + ( 16 x 16)
AB = 20
.
Q.18 Meethapur works on a barter system. Each person there loves different sweets. Mukund is
willing to exchange 2 toffees for a lollypop and 3 ice creams for a toffee. Ranjana is willing to exchange
one ice cream for 2 lollypops and 3 toffees for a lollypop. Mukund and Ranjana don’t know each other,
but both of them know Sita who loves toffees. Sita has one lollypop. She can meet Ranjana and
Mukund only once (not necessarily in that order). What is the maximum number of toffees that Sita
can get?
Ans Let us assume that Sita goes to Mukund first ,
She exchanges her 1 lollypop for 2 toffees
With these 2 toffees she get 6 ice creams from Mukund.(2x3)
Now she goes to Ranjana having 6 icecreams in hand
She gets 12 lollypops for that.(6x2)
She again exchange 12 lollypops and gets 36 toffees (12x3)
now assume that Sita goes to Ranjana first than
She exchanges her 1 lollypop for 3 toffees only.
Then she goes to Mukund , she can get only icecreams and lollypops .& no more toffees .
( as the condition is that she can meet them once only)
ans is maximum 36 toffees she can get.
Q19. Atul has nine cats that always fight with each other. He puts all of them into a square box.
What is the least number of square partitions he must use to keep all the cats separated from each other? You may use any size of square partitions.
Ans 2 squares , one placed diagonally as shown.
OR 4 squares as shown
Q 20 How many differences are there in the two images shown below?
Ans There are 10 differences
Check the difference for
- Change in colour
- Change in pattern
- Missing object
- Additional object
* There are 20 questions in SECTION A .
* Try to solve this section within 40 minutes.
* As there is no negative marking try to attempt all questions.
